The word hyperbola is a Greek word that means excessive. It is a group of all those points, the difference of whose distances from two fixed points is always same or constant. The fixed points are called as the foci(foci is plural for the word focus.) A hyperbola has two curves that are known as its arms or branches. These branches continue up to infinity.
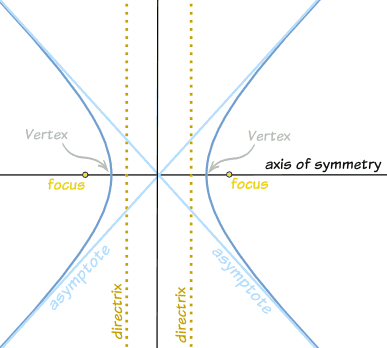
Table of Contents
Facts About Hyperbola:
- So unlike an ellipse, a hyperbola is an open figure.
- It may be symmetric about either of the x-axis or y-axis.
- If a it is symmetric about x-axis, then its equation is of the form: x2/a2 – y2/b2 = 1
- If it is symmetric about the y-axis, then its equation is of the form: y2/a2 – x2/b2 = 1
- The axis about which the hyperbola is symmetric is called the major axis of it , and the other one is called the minor axis.
- The major axis is also known as transverse axis and the minor axis as the conjugate axis.
- Half of major axis is the semi-major axis or semi-transverse axis, and half of minor axis is semi-minor axis or semi-conjugate axis. In the equations of the hyperbola, a is the length of semi-major axis, and b is the length of the semi-minor axis. Therefore the length of the major or transverse axis is 2 x a, and the length of minor axis or conjugate axis is 2 x b. It has two focus. The difference of the distances of any point on the hyperbola from the two focus is always constant. The distance of focus from the centre of it is represented by c. It is known as semi-focal length.
Relation between c(the distance of focus and center) , a(length of semi transverse axis) and b(length of semi conjugate axis) :c2 = a2 + b2.
The ratio of c(the distance of focus and centre) to a(length of semi-transverse axis) is constant and is called as eccentricity. It is represented by e. The value of e for a hyperbola is greater than 1. A line that passes through the focus and intersects it at two points is called as latus rectum. It is perpendicular to the major axis of it.
Some important formulae:
• Eccentricity = c/a.
• Relation between c , a and b is c2 = a2 + b2.
• Length of major axis = 2 x a.
• Length of minor axis = 2 x b.
• Length of latus rectum = 2b2/a.
As we know that the word hyperbola means excessive. This name is chosen because an ellipse has eccentricity less than 1, it has an eccentricity greater than 1, and a parabola has an eccentricity equals to 1.
Hyperbola in Nature (Real Life):

The difference between a parabola, a hyperbola and a catenary curve Equations:
The equations of the four types of conic sections are as follows.
- Circle- x2+y2=1
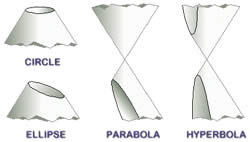
- Ellipse- x2/a2+ y2/b2= 1
- Parabola- y2=4ax
- Hyperbola- x2/a2– y2/b2= 1
If you have any Question, you may write in comment section below










